The ancients and mathematics

—Numbers enabled the construction of complex urbanised societies, which mobilised many resources.—

If there was one discipline that attracted the interest of all ancient societies, it was mathematics. Egypt, Babylon, India, Greece, China and Mesoamerica showed that there was great diversity around the development of mathematics. This is shown by the problems that were of interest in each case, as well as by the variety of types of predominant reasoning, the relationship established between mathematics and other inquiries and the value that these had in each society. Without mathematics, without numbers, civilisation, meaning ordering and controlling resources, a monetary economy that allowed for accounting, surveying, the census and the taxation system, would not have been possible.

Clay Tablet, with geometric and algebraic mathematics, similar to Euclidean mathematics (Iraq Museum, Tell Harmal, 2003-1595 BC). Wikipedia.

Ancient Babylon was the setting for the earliest known studies of mathematics. The thousands of clay tablets engraved with the characteristic cuneiform script reveal that a significant group of scribes received training in schools, where they were trained to solve many issues of interest to the State, particularly legal and financial disputes, trade, laws and agriculture (finding out the area of fields, the shape of irrigation canals and the structures of reservoirs). They memorised long multiplication and division tables. Clay and reeds, styli with a triangular tip, used as tools for writing determined the numerical systems. These circumstances forced them to count in blocks of sixty, a peculiarity that conditioned and influenced the rest of later geometry. Mathematics underwent a clear evolution throughout Mesopotamian history, until it reached a way of thinking in which matter was detached from the practical problem that it originated from (the calculation of payments, distribution of an inheritance, measuring of fields etc.) to conform to an internal dynamic. In addition to arithmetic and geometric progressions, there were linear, quadratic, cubic and exponential equations and equations of other types. Systems of various equations were solved and logarithms were used, among other mathematical processes.

In the case of Egypt, we find less development and much less available information. There are only five Egyptian papyri, such as those of the Rhind (1650 BC), Moscow (1890 AC) and Berlin (1800 AC). However, it can be pointed out that there was an interest in explaining the methods and problems of arithmetic and geometry to students, for example, linear equations, arithmetic and geometric series, prime numbers and the solution of second-degree algebraic equations. Unlike the Babylonians, the Egyptians opted for a decimal, non-positional system, initially developed with hieroglyphic pictograms and individual symbols. With the introduction of papyrus and ink, it was possible to simplify and accelerate the writing process, which was more italic (hieratic) and would eventually lead to the demotic. Thus, the set of symbols was considerably reduced as was the need for memorisation. Nevertheless, these two great civilisations developed an extraordinary and complex administration and system of government that would have been unthinkable without a method of writing and all that stemmed from it. All this knowledge was essential for the later advances in mathematics in the Greek world.

Detail of mathematical aspects of the Rhind papyrus (British Museum, EA10057, Thebes, c. 1550 BC). Wikipedia.

Of all the Hellenistic contributions, it has been stated that the most impressive and inspiring of all was mathematics. Not only due to the achievements made, but also because they were achieved in an incredibly short period of time. That said, the Greeks changed their mathematical perspective when they turned to abstract geometric knowledge and formal methods of inference and proof. Without doubt, one of the best known pioneers was Pythagoras (569-475 BC). He founded a philosophical and religious school in southern Italy with many followers. They called themselves matematikoi; both men and women, they lived in the heart of this society on a permanent basis, had no personal possessions and were vegetarians.  Pythagoreans were not interested in ‘formulating or solving mathematical problems’, nor did ‘open problems’ exist for them in the traditional sense of the term. Pythagoras’ interest was in ‘the principles’ of mathematics, ‘the concept of number’, ‘the concept of the triangle’ (or other geometric figures), and the abstract idea of ‘proof’. Pythagoras was above all an expert on topics such as immortality, reincarnation of the soul and its destiny after death, religious rituals and self-control and discipline.  He was the founder of a way of life and thinking that was supported by many. Thus, Plato was a follower of Pythagoreanism. In fact, for him, everything connecting the cosmos was a geometric proportion. In contrast, Aristotle marked a difference between mathematics and nature; geometry did not exhaust reality at all.

Pythagoreans celebrating the dawn (Fyodor Bronnikov, 1869). Wikipedia.

Understanding what mathematics was, the meaning that was given to them and what their use was for the people of the ancient world is a challenge for the way of understanding these matters in the present. On the other hand, there were also various ways of understanding mathematics in Antiquity. For example, when the ‘shu’ (number) was referred to in the Chinese world, the semantic field that this term covered was wider than its current meaning. The ‘shushu’, or study of the ‘shu’ constituted a much wider approach than the use of numbers and quantities. In the Chinese context, many mathematical treatises were developed in its classical period, about one hundred years before and after the birth of Christ which marked the most common way of establishing the period in Europe. They used a system of arithmetic notation which was fairly similar to the European one, with symbols whose value was given by their position and with a decimal base. In contrast, their methods of calculation were carried out with ruled tablets. The Chinese designed a system which allowed them to connect very different phenomena in a very organised way; the understanding reached was associative, not explanatory. Each object formed part of a network of relationships and held a place within a framework of global interpretation. Chinese mathematicians wanted to find the essential elements that could unite all subjects (‘ganji’). For Chinese culture, mathematics was a source of cohesion, harmony and unity.

Title page of Euclid’s Geometrical Elements, published by Jacobus Kresa in 1689. Wikipedia.

Around 300 AC, the most outstanding moment of ancient science in the Mediterranean area took place. A group of Hellenistic mathematicians sought to separate themselves from the tradition of the Egyptian and Babylonian worlds, and distance themselves from other groups of intellectuals, such as philosophers and sophists, who were also interested in mathematics. They never formed a professional body that would make a living from their mathematical skills. In fact, they carried out very diverse activities and many were soldiers, merchants, politicians and philosophers. They seem to have worked alone and never created a school. Athens was an exception, as there they did form a group to work or at least shared problems. In addition, other acquaintances in well-known Alexandria would create a school. Euclid, Archimedes and Apollonius are certainly the most prominent figures. They were of different origins but trained in the Egyptian city. Nevertheless, it is also true that there was a large group of lesser-known mathematicians, such as Eratosthenes, Nicomedes, Diocles, Hipsicles or Zenodorus, and each had their own original contributions.

The well-known work of the first, Euclid, contrasts with how little we know about his life. His Elements were the most successful mathematical work of all time: it replaced many others and none could discard it. It was translated into many languages and with the printing press more than a thousand editions were made; it was used as a textbook in many schools and universities. Taken to the East by the Jesuit missions, it was translated into Chinese at the beginning of the seventeenth century. Euclid practised and taught rigorous mathematics, far from any practical application, at the Museum of Alexandria. His thirteen books deal with arithmetic and algebra but he was essentially recognised for his geometry, which was not surpassed until the nineteenth century. Although Archimedes followed Euclidean geometry, he gave it another perspective.

The application of mathematics in the study of nature is very clear in aspects such as astronomy, optics and principles such as scales or the lever. In all these areas, Hellenistic mathematicians provided elegant models that were of great influence. Regarding the first instance, the ground was prepared for a model that would be successfully reach the so-called “Scientific Revolution” over three generations: Eudoxus created the first geometric model of planetary motion; Heraclides, Aristarchus and Hipparchus improved the understanding of the cosmos and Ptolemy would brilliantly complete the cosmological model that lasted 1500 years.

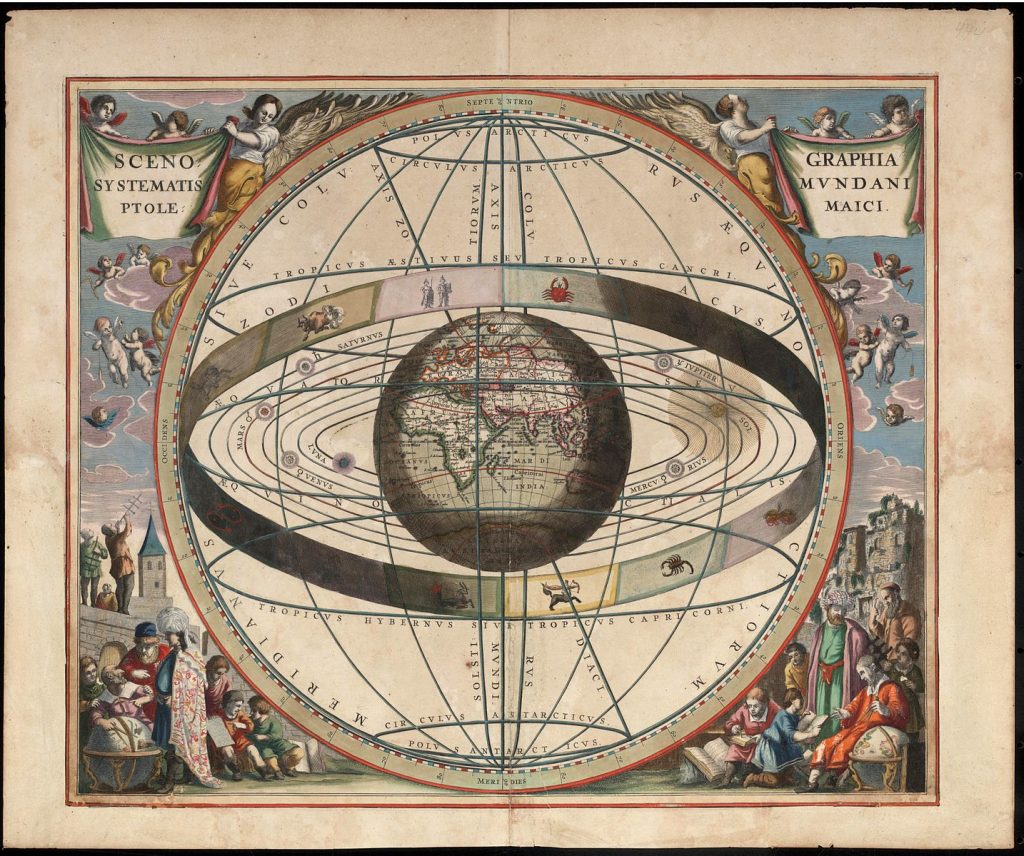
Graphic representation of Ptolemy’s world view with the signs of the zodiac, the solar system and the Earth in the centre (Andreas Cellarius, Harmonia Macrocosmica, 1660/61). Wikimedia.

On the eve of the fall of the Roman Empire, in the fifth century, mathematical studies were characterised by the use of meticulous commentaries on works that had been key in earlier times. Authors such as Archimedes, Euclid or Apollonius went through this process of commenting in order to clarify definitions, attach some demonstrations or clarify the relationship between theorems. Some works of this type came to offer new results. Something similar happened in the Hellenistic area. In that century, some treatises were still being written but, with the triumph of Christianity, Platonism fell into disrepute and, in 529, Emperor Justinian would close the Academy. In the last days of the Alexandrian school, Pappus, Theon of Alexandria and his daughter Hypatia stand out for the commentaries on the Almagest and the works of Euclid, and the commentaries on Apollonius and Diophantus, respectively, works that have been lost today.

Carmel Ferragud
IILP-UV

How to cite this paper:
Ferragud, Carmel. The ancients and mathematics. Sabers en acció, 2020-11-13. https://sabersenaccio.iec.cat/en/the-ancients-and-mathematics/.

Find out more

You can find further information with the bibliography and available resources.